
The very expensive college system exaggerates risks we did not have to consider in past generations. Having a clear understanding of college risks leads to better outcomes.
I have been in risk management for most of my career. This article provides essential information on using a risk manager’s perspective to make a great college decision.
About the author: Jeff Hulett is a career banker, data scientist, and choice architect. Jeff has held banking and consulting leadership roles at Wells Fargo, Citibank, KPMG, and IBM. Today, Jeff is an executive with the Definitive Companies. He teaches personal finance at James Madison University and provides personal finance seminars. Check out his book -- Making Choices, Making Money: Your Guide to Making Confident Financial Decisions -- at jeffhulett.com.

Please check out Jeff's YouTube channel for the presentation of this article: How would a risk manager advise a college decision-maker?
Warren Buffett is one of the most successful investors in history. Mr. Buffett commented:
“Price is what you pay, value is what you get.”
This is a risk manager’s and an investor’s perspective of the world. Understanding the value of a good, like a college education, is very different than determining the price of that same good. Understanding value is challenging. Especially in the case of college, the value will be realized decades in the future, whereas the cost is committed to today. My chief aim is to help you make the best college decision, by optimizing the value-to-cost tradeoff.
Most investors look to arbitrage the value-to-cost tradeoff. This means that when an investor is provided with a group of similar investment choices, they will seek to pay as little as possible for similar value. [xv] Also, risk managers and investors like Warren Buffett consider both risks and return for investments. While many college pundits suggest “College is a good investment” they rarely cite the risk side of the college equation.
College like a mutual fund investment: To an investor, not considering risk is like buying a mutual fund based on historical return only, without considering the risks of the same investment. Risk ratings are regularly provided for financial investments. [xvi] It does seem strange, given investors universally consider both risk and return when making mutual funds or other financial investments, that many downplay the risks of the college investment. On the other hand, perhaps it is not so strange that college risk is downplayed, given the two "selective college narrative" support points offered in the "Our college hope" article. Either way, given the employer's view, this suggests a rational person may want to pay as little as possible for a good GPA. But this still leaves open the question of college risk. Is the risk meaningful?
Is it worth taking a risk paying a higher college cost as compared to a lower-cost college alternative? The short answer is, on average:
Probably Not.
First, to explore college risk, we need to discuss what risk is. In the traditional risk world, we define risk by two essential features – these risk features are called “frequency” and “severity.”
Frequency is defined as → What is the likelihood a risk will occur? and if it does,
Severity is defined as → How much will the risk cost us?
Frequency risk: In the context of college, frequency risk relates to the probability that a high school student, at the time the college acceptance decision is made, will not achieve the desired outcome in the future. The following graphic shows several frequency risk outcomes, based on increasing frequency risk significance [xvii]. This is based on U.S. aggregate data from The Wall Street Journal and Pew Research.

This pie chart shows outcomes 10 years after the student enters college. Notice only about 30% of high school students achieve a college degree and obtain a job requiring a college degree. Those are the green and light green colored slices. Even within the 30% group, only about 9% of the population – which is the darker green slice - can get a degree, a job requiring a college degree, and not have student debt. The remaining approximately 70% have progressively higher frequency risk-impacted outcomes, such as not graduating, not getting a job requiring a college degree, and defaulting on a loan. The college investment payoff relates to higher-paying jobs and higher-paying jobs often require college degrees. Based on this view, four-year colleges carry significant frequency risk.
Is the BLS fooling us? If there is so much college risk, why does the BLS graphic show such a different perspective?
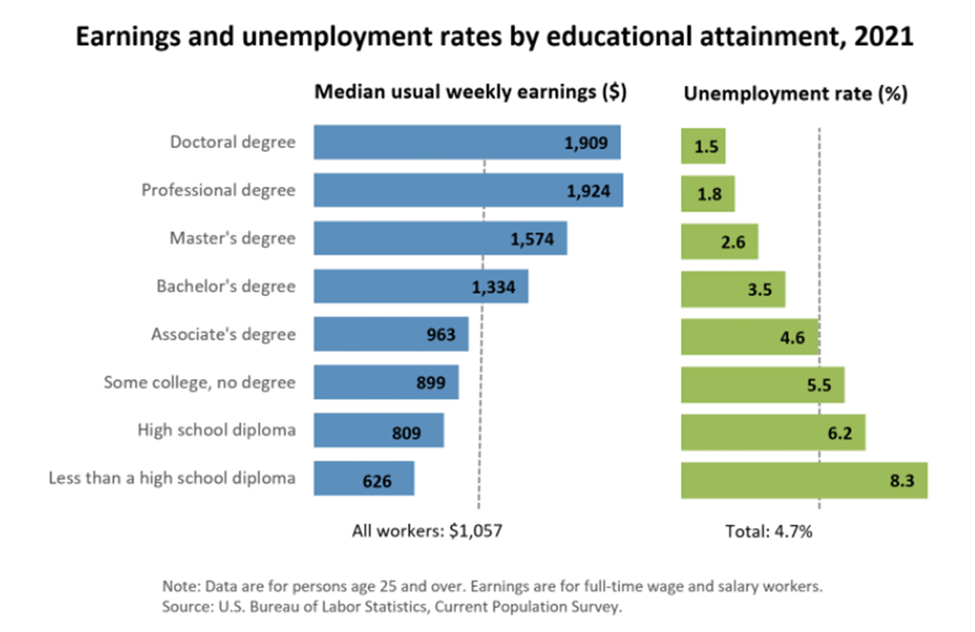
This is the “College Pays” graphic from the U.S. Bureau of Labor Statistics (BLS). There is such an interesting inconsistency between the frequency risk graphic and the BLS narrative. The reason the BLS graphic is so different is that they only look at return-based education outcomes. This is a ‘cherry-picked’ outcome reported long after the decision to go to college is made. Our analysis from the Wall Street Journal and Pew Research uses a vintage technique (aka, static pool) that considers future outcomes based on when a high school student originally makes the college choice. The vintage approach is the standard decision analytics approach for risk managers and others to make a good decision at the time one has multiple decision alternatives. [xviii] Keeping in mind, “Not Yet” is a valid option. Also, ironically, the BLS average income analysis only considers gross income. Collectively, United States citizens have over $1.75 Trillion outstanding in student loans. [xix] To properly evaluate, the BLS could net the average gross income against the higher debt service needed for many with higher educational attainment.
Severity risk: Now, let’s switch gears to the other risk feature called severity. Severity addresses “how bad the risk could be.” For this, we use a long-term wealth substitution approach. This approach compares the difference between:
A "Savvy School" – this is where acquiring a four-year college education that costs less, so no college debt is required. This is compared to
A "Dream School" – this is where acquiring a four-year college education where the average debt is required.
Savvy school could be achieved in many ways. It could be a public university or an online college. It could include scholarships and grants. A savvy school could include starting with community college or working. The point is that the Savvy school student figures out how to get college costs down!
According to the Education Data Initiative, average monthly student loan payments are about $400 and the average loan takes 20 years to pay off. For each young person with the average student loan, the long-term financial impact of paying back a student loan instead of saving for retirement is over $3.5 million. [xx] By substitution, this means a new college graduate who recently started loan repayment is paying $400 a month in loan payments instead of placing $400 a month into a retirement account. This is the negative impact of delaying getting on the time value of money wealth-building ladder.
This begs the question:
"Is taking on debt for a more expensive college worth reducing retirement by $3.5mm - compared to a college option not requiring debt?"
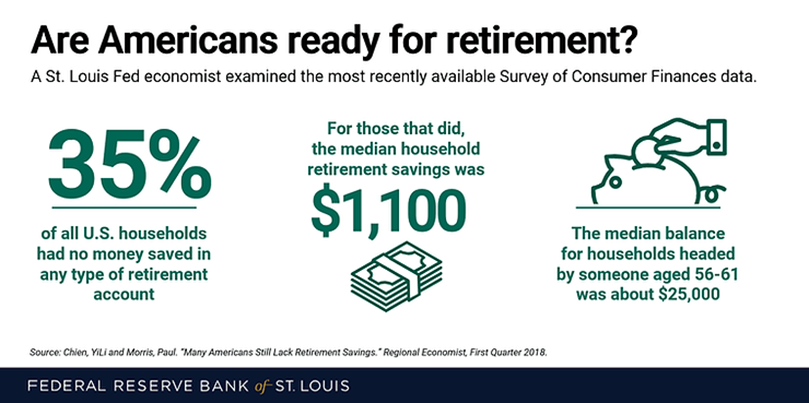
To help answer this question, in our notes is the U.S. Federal Reserve’s perspective on the tragically low level of U.S. retirement savings. [xxi]

This analysis shows the negative long-term financial effect of substituting college debt for an investment like a 401(k) retirement account.
To summarize the risk managers perspective:
The frequency risk, based on the average high school student starting college is HIGH. Approximately 70% of those starting college will not achieve the desired outcome including a college degree and a job requiring a college degree. Only 9% reach the desired outcome without college debt.
The severity risk, based on the financial outcome of substituting debt service for a retirement contribution is HIGH. The average loan payment will reduce retirement savings by $3.5 million.
Thus, many Americans starting college do not achieve the desired college outcome. This inability to achieve the desired outcome likely impacts long-term financial capacity and retirement savings.
Now, you know something very valuable. This risk information is essential to making a great college decision. It should NOT scare you away from college. It should help focus you on making the best college decision.
When the college decision is made, the high school student will know if they need loans or not. [xxii] Thus, they are aware of whether they will start in the darker green or lighter green slice. If they are light green, they already know the average severity will cost them $3.5mm for retirement. At this point, the key is:
Minimizing the chance their frequency risk slice gets hotter in color. Historically, almost 70% of Americans do transition to a hotter slice.
Keep the slice as dark green as possible by minimizing debt accumulated for college.
The goal is to STAY GREEN!
To this end, it is important to overlay the employer's perspective to help manage the risk. We discussed this in the article called “What do employers think of college graduates?”
To connect the dots, we can
·Reduce college risk,
·By achieving a major and GPA that maximizes the likelihood of a positive college outcome.
In our article “What do employers think of college graduates?” we discussed the importance of study habits and other essentials for reducing college risk.
Admittedly, everyone’s situation is different. Think of these risk management rules of thumb to help guide your individual story.
Keeping college costs down will help manage severity. This is true even if the student does not need loans for college. One should always be a good steward of resources.
My book provides resources, like apps, to help you make the best college decision.
Notes
[xv] For more information on the college decision process as related to economic discrimination and price discrimination, please see the article:
Hulett, Why buying a car is so similar to buying a private college education, The Curiosity Vine, 2022
[xvi] Editors, Morningstar Risk Rating, Corporate Finance Institute, 2022
A position or score that is given to publicly-traded ETFs (exchange-traded funds) or mutual funds.
[xvii] Hulett, The College Decision: Proceed at your own risk, The Curiosity Vine, 2021
[xviii] Vintage analysis is commonly used in banking and financial services-related risk analysis. More generally, vintage analysis is also known as static pool analysis. A static pool analysis is commonly used to measure the effects of a randomized controlled trial or "RCT." RCT is commonly used to differentiate between multiple groups with scientifically controlled variations. The following are resources for vintage analysis in financial services and RCT in scientific studies:
Credit Union Examiner guidance, Static Pool Analysis: Evaluation of Loan Data and Projections of Performance, National Credit Union Association, 2006
Cantarero-Villanueva, et al, The Effectiveness of a Deep Water Aquatic Exercise Program in Cancer-Related Fatigue in Breast Cancer Survivors: A Randomized Controlled Trial, Archives of Physical Medicine and Rehabilitation, 2013
[xix] Hahn, Traver, 2022 Student Loan Debt Statistics: Average Student Loan Debt, Forbes Advisor, 2022
[xx] Financial Model developed by the author, assumes a 10% annual pre-tax yield, beginning at age 22 and ending at age 67. Loan data and term assumptions are from the Education Data Initiative, https://educationdata.org/average-student-loan-payment
Another approach to describing the same model is to consider retirement outcomes based on delayed savings impact. The following graphic shows the "tyranny of the exponential function." Notice that just delaying 5 years from age 22 to age 27 decreases potential retirement value by 40%.

Even if one is fortunate to not need a loan, severity risks are resident in the cost of a college education. If the family is paying for an education, a higher cost of college will reduce the estate or other multiple generational-based inheritances. See our article where we model a $4.7 million impact example. This is based on retirement outcomes without student loan debt.
Hulett, The College Stoic: The Stoic's Arbitrage and making a great college decision, The Curiosity Vine, 2022
[xxi] Our begged question is whether $3.5mm is meaningful for retirement. Based on current estimates, a vast minority of Americans are properly saving for retirement. Please see this Federal Reserve infographic. As such, a $3.5mm substitution for college debt would be very impactful to the vast majority of Americans.
[xxii] As part of the Federal Student lending process, a high schooler and their family determine loan qualification via an application known as the FAFSA (Free Application for Federal Student Aid). The aid eligibility will be provided in the form of SAR (Student Aid Report). The student loan determination will be made by the individual colleges, based on their cost of attendance.
Editors, Complete the FAFSA® Form, U.S. Department of Education, Federal Student Aid website, 2022

Comments